Trigonometric Identities
The Law of Sines
The law of sines can be used to find unknown angles and sides in any triangle.Learning Objectives
Use the law of sines to solve problems with triangles of any configuration, as well as to transform trigonometric expressionsKey Takeaways
Key Points
- The law of sines is used to find the measurements of all three angles and all three sides in a triangle.
- The law of sines states that the following proportions are equal: , where and are angles and , , and are the lengths of the sides opposite them, respectively.
- Any pair of applicable ratios from the law of sines formula can be used to solve for an unknown angle or side length in any triangle.
Key Terms
- oblique triangle: A three-sided shape that does not contain a angle.
Solving Triangles Using The Law of Sines
Previous concepts explained how to use trigonometry to find the measures of the angles and sides of right triangles. We will now discuss the law of sines, which allows us to solve for the angles and side lengths of any triangle. A right triangle contains a angle, while any other triangle is an oblique triangle. Solving an oblique triangle means finding the measurements of all three angles and all three sides. The law of sines states that: where and are angles and , , and are the lengths of the sides opposite them, respectively. Oblique triangle: The sides of this oblique triangle are labeled a, b, and c, and the angles corresponding to them are labeled , , and .
Oblique triangle: The sides of this oblique triangle are labeled a, b, and c, and the angles corresponding to them are labeled , , and .Note the standard way of labeling triangles: angle (alpha) is opposite side ; angle (beta) is opposite side ; and angle (gamma) is opposite side .
To solve an oblique triangle, use any pair of applicable ratios from the law of sines formula. While calculating angles and sides, be sure to carry the exact values through to the final answer.Example
Solve the triangle shown in the figure, with final answers rounded to the nearest tenth. Oblique triangle with unknown sides and angles: In this triangle, , , and . The angle (corresponding to side ) and the side-lengths and (corresponding to angles and , respectively) are unknown.
Oblique triangle with unknown sides and angles: In this triangle, , , and . The angle (corresponding to side ) and the side-lengths and (corresponding to angles and , respectively) are unknown.First, notice that two of the three angles are already identified. We can subtract these from to find the measure of angle :
\begin{align} \beta &= 180^{\circ} - 50^{\circ} - 30^{\circ} \\ &= 100^{\circ} \end{align} To find an unknown side, we need to know the corresponding angle and a known ratio. We know that angle and its corresponding side is . Let's find the length of side , which corresponds to angle . We can use the following proportion from the law of sines: Multiply both sides by : Multiply by the reciprocal of to isolate : Solving this with a calculator, we obtain: The last unknown side is , and we will follow a similar process for this. Set up another proportion: Solve for : \displaystyle{ \begin{align} \frac{b \cdot \sin{\left(50^{\circ}\right)}}{10} &= \sin{\left(100^{\circ}\right)} \\ b \cdot \sin{\left(50^{\circ}\right)} &= 10 \cdot \sin{\left(100^{\circ}\right)} \\ b &= \frac{10 \cdot \sin{\left(100^{\circ}\right)}}{\sin{\left(50^{\circ}\right)}} \\ b &\approx 12.9 \end{align} } Therefore, the complete set of sides and angles is:The Law of Cosines
The law of cosines can be used to solve for angles and sides of a triangle in cases where other laws do not apply.Learning Objectives
Use the law of cosines to solve problems with triangles of any configuration, as well as to transform trigonometric expressionsKey Takeaways
Key Points
- The law of cosines can be used to find the measurements of angles and sides of a triangle in cases where the law of sines cannot be applied, such as for triangles with no known angles.
- The law of cosines states that the square of any side of a triangle is equal to the sum of the squares of the other two sides minus twice the product of the other two sides and the cosine of the included angle (for example, ).
- The law of cosines has noted similarities to the Pythagorean theorem, from which it is derived.
Key Terms
- Pythagorean theorem: A fundamental relation among the sides of a right triangle, which states that where is the hypotenuse, and and are the lengths of the other two sides.
Using The Law of Cosines
In some cases, we may not have enough information to apply the Law of Sines to find unknown angles and sides in a triangle. For example, consider a triangle where all three sides are known, but no angle values are known. In such cases, there is not enough information to use the Law of Sines. The Law of Cosines is useful for: 1) computing the third side of a triangle when two sides and their enclosed angle are known, and 2) computing the angles of a triangle if only the three sides are known. The Law of Cosines defines the relationship among angle measurements and side lengths in oblique triangles. Three formulas make up the Law of Cosines. At first glance, the formulas may appear complicated because they include many variables. However, once the pattern is understood, the Law of Cosines is easier to work with than many formulas at this mathematical level. The Law of Cosines states that the square of any side of a triangle is equal to the sum of the squares of the other two sides minus twice the product of the other two sides and the cosine of the included angle. For triangles labeled with angles , , and , and opposite corresponding sides , , and , respectively, the Law of Cosines is given as three equations: \begin{align} a^2 &= b^2 + c^2 - 2bc\cos\alpha \\ b^2 &= a^2 + c^2 - 2ac\cos\beta \\ c^2 &= a^2 + b^2 - 2ab\cos\gamma \end{align} Oblique triangle (no right angle): An oblique triangle, with angles , , and , and opposite corresponding sides , , and .
Oblique triangle (no right angle): An oblique triangle, with angles , , and , and opposite corresponding sides , , and .To solve for a missing side measurement, the corresponding opposite angle measure is needed. When solving for an angle, the lengths of all of the sides are needed. Notice that each formula for the Law of Cosines can be rearranged to solve for the angle. For example, to solve for the angle , the first formula can be rewritten as:
The Law of Cosines is a more general form of the Pythagorean theorem, which holds only for right triangles. Notice that if any angle in the triangle is a right angle (of measure ), then , and the last term in the Law of Cosines cancels. Thus, for right triangles, the Law of Cosines reduces to the Pythagorean theorem:Example
Find the length of the unknown side of the triangle in the figure shown, and round the value to the nearest tenth. Example oblique triangle: This oblique triangle has known side lengths and , and known angle .
Example oblique triangle: This oblique triangle has known side lengths and , and known angle .First, make note of what is given: two sides and the angle between them. This is not enough information to solve the problem using the Law of Sines, but we have the information needed to apply the Law of Cosines.
We can use the Law of Cosines to solve for side , since we know the measurement of the opposite angle . The appropriate formula is: Substitute the values of , , and from the given triangle: From the unit circle, we find that . Substitute this into the formula and evaluate: \displaystyle{ \begin{align} b^2 &= 10^2 + 12^2 - 2\left(10\right)\left(12\right)\left(\frac{\sqrt{3}}{2}\right) \\ b^2 &= 100 + 144 - 240\frac{\sqrt{3}}{2} \\ b^2 &= 244 - 120\sqrt{3} \\ b &= \sqrt{244 - 120\sqrt{3}} \\ b &\approx 6.0 \end{align} } Note that we now have enough information that we could use the Law of Sines to solve for the unknown angles and in the triangle.Pythagorean Identities
The Pythagorean identities are useful in simplifying expressions with trigonometric functions.Learning Objectives
Connect the trigonometric functions to the Pythagorean Theorem in order to derive the Pythagorean identitiesKey Takeaways
Key Points
- The Pythagorean identities are derived from the Pythagorean theorem, and describe the relationship between sine and cosine on the unit circle.
- The three identities are , , and .
- The Pythagorean identities can be used to simplify problems by transforming trigonometric expressions, or writing them in terms of other trigonometric functions.
Deriving the Pythagorean Identities
Using the definitions of sine and cosine, we will learn how they relate to each other and the unit circle. For any point on the unit circle, We can see how this relates to the Pythagorean theorem, For a triangle drawn inside a unit circle, the length of the hypotenuse of the triangle is equal to the radius of the circle, which is . The lengths of the sides of the triangle are and . The Pythagorean identity on a unit circle: For a triangle drawn inside a unit circle, the length of the hypotenuse is equal to the radius of the circle. The triangle sides have lengths and .
The Pythagorean identity on a unit circle: For a triangle drawn inside a unit circle, the length of the hypotenuse is equal to the radius of the circle. The triangle sides have lengths and .Because and on the unit circle, we can substitute for and to get the Pythagorean identity:
which is true for any real number . We can use the Pythagorean identity to find the cosine of an angle if we know the sine, or vice versa. However, because the equation yields two solutions, we need additional knowledge of the angle to choose the solution with the correct sign. If we know the quadrant where the angle is, we can easily choose the correct solution. Additional identities can be derived from the Pythagorean identity . For example, if we divide the identity by , we have the following identity: \displaystyle{ \begin{align} \cos^2 t + \sin^2 t &= 1 \\ \left(\frac{\cos^2 t}{\cos^2 t}\right) + \left(\frac{\sin^2 t}{\cos^2 t}\right) &= \left(\frac{1}{\cos^2 t}\right) \\ 1 + \tan^2 t &= \sec^2 t \end{align} } Likewise, if we divide the identity by , we have the following: \displaystyle{ \begin{align} \cos^2 t + \sin^2 t &= 1 \\ \left(\frac{\cos^2 t}{\sin^2 t}\right) + \left(\frac{\sin^2 t}{\sin^2 t}\right) &= \left(\frac{1}{\sin^2 t}\right) \\ 1 + \cot^2 t &= \csc^2 t \end{align} } These equations are also called Pythagorean trigonometric identities.Summary
We have derived three Pythagorean identities:Applications
The Pythagorean identities can be used to simplify problems by transforming trigonometric expressions. In expressions with multiple trigonometric functions, the Pythagorean identities can be used to substitute and simplify the expression. For example, consider the following: Let's try to simplify this. We know that cosecant is the reciprocal function of sine. In other words, we can say We can also recognize that , which is simply the Pythagorean identity rearranged. Therefore, we can rewrite the expression in terms of sine: The sine functions cancel and this simplifies to , so we have: \displaystyle{ \begin{align} \left(1 - \cos^2 t\right) \csc^2 t &= \left(\sin^2 t\right)\left(\frac{1}{\sin^2 t}\right) \\ &= 1 \end{align} } When simplifying expressions with trigonometric functions, it is helpful to look for ways to use the Pythagorean identities to cancel terms. The problem below provides another helpful example.Example
Simplify the following expression: This looks like a very complicated problem, but let's look for things we can cancel. First, notice that we have both and , and group them together: We can factor out the : We can now substitute for , applying the Pythagorean identity: Look at the remaining terms in the expression. Recall that one of the Pythagorean identities states . This can be rearranged to . Substituting this into the expression, we have: \displaystyle{ \begin{align} 5 + \sec^2 t - 1 - \tan^2 t &= 5 + \left(1 + \tan^2 t\right) - 1 - \tan^2 t \\ &= 5 + 1 - 1 + \tan^2 t - \tan^2 t \\ &= 5 + \tan^2 t - \tan^2 t \\ &= 5 \end{align} } The expression simplifies to .Angle Addition and Subtraction Formulae
Trigonometric expressions can be simplified using special angles and a set of formulae for adding and subtracting angles.Learning Objectives
Simplify trigonometric expressions using the angle addition and subtraction formulae.Key Takeaways
Key Points
- The formulae for addition and subtraction of angles in trigonometric expressions allow us to find the sine, cosine, or tangent of a given angle if we can break it up into the sum or difference of two of the special angles.
- The formulae for cosine are: and .
- The formulae for sine are: and .
- The formulae for tangent are: and .
Key Terms
- distance formula: An equation to find the length of the line between two points on the -plane. The length of the line between and is given by: .
Deriving the Angle Addition and Subtraction Formulae
Finding the exact value of the sine, cosine, or tangent of an angle is often easier if we can rewrite the given angle in terms of two angles that have known trigonometric values. We can use the special angles, which we can review in the unit circle shown below. Unit circle: The unit circle with the values for sine and cosine displayed for special angles.
Unit circle: The unit circle with the values for sine and cosine displayed for special angles.There are formulae for the addition and subtraction of angles within each of the trigonometric functions. These allow us to find the trigonometric function of a given angle if we can break it up into the sum or difference of two of the special angles.
To see how these formulae are derived, we can place points on a diagram of a unit circle. Suppose that the angle we want to find a trigonometric function for is the one formed by the point , which measures an angle . The angle formed by and a point on the positive -axis is the same as the angle formed between two special angles, which are denoted by and . Point is at an angle from the positive -axis with coordinates , and point is at an angle of from the positive -axis with coordinates . The angles are equal, and so the distance between points and is the same as between points and . Relationships between angles on the unit circle: The formulae for adding and subtracting angles are derived from the relationships between angles on the unit circle.
Relationships between angles on the unit circle: The formulae for adding and subtracting angles are derived from the relationships between angles on the unit circle.Using the equivalence of these distances and the distance formula:
it is possible to derive a number of relationships between the angles. We can derive the following six formulae. The formulae for cosine are: \begin{align} \cos(\alpha + \beta) &= \cos \alpha \cos \beta - \sin \alpha \sin \beta \\ \cos(\alpha - \beta) &= \cos \alpha \cos \beta + \sin \alpha \sin \beta \end{align} The formulae for sine are: \begin{align} \sin(\alpha + \beta) &= \sin \alpha \cos \beta + \cos \alpha \sin \beta \\ \sin(\alpha - \beta) &= \sin \alpha \cos \beta - \cos \alpha \sin \beta \end{align} The formulae for tangent are: \displaystyle{ \begin{align} \tan(\alpha + \beta) &= \frac{ \tan \alpha + \tan \beta}{1 - \tan \alpha \tan \beta} \\ \tan(\alpha - \beta) &= \frac{ \tan \alpha - \tan \beta}{1 + \tan \alpha \tan \beta} \end{align} } These are useful for finding angles that can be derived by adding or subtracting special angles. For example, consider the angle , which is the sum of two special angles: and . The trigonometric functions of any such angle can be found.Example
Using the formula for the cosine of the difference of two angles, find the exact value of . Apply the formula : Substitute the values of the trigonometric functions from the unit circle: Simplify: \displaystyle{ \begin{align} \cos{\left(\frac{5\pi}{4} - \frac{\pi}{6}\right)} &= -\frac{\sqrt{6}}{4} -\frac{\sqrt{2}}{4} \\ \cos{\left(\frac{5\pi}{4} - \frac{\pi}{6}\right)} &= -\frac{\sqrt{6}-\sqrt{2}}{4} \end{align} }Example
Find the exact value of . First, notice that is the difference of two special angles: . We can thus apply the formula for sine of the difference of two angles: . Substitute and into the formula: Substitute the values of the trigonometric functions from the unit circle: Simplify: \displaystyle{ \begin{align} \sin{\left(45^{\circ} - 30^{\circ}\right)} &= \frac{\sqrt{6}}{4} - \frac{\sqrt{2}}{4} \\ \sin{\left(45^{\circ} - 30^{\circ}\right)} &= \frac{\sqrt{6} - \sqrt{2}}{4} \end{align} } Thus, we have:Double and Half Angle Formulae
Trigonometric expressions can be simplified by applying the double- and half-angle formulae.Learning Objectives
Simplify trigonometric expressions using the double and half angle formulaeKey Takeaways
Key Points
- The double-angle formulae are a special case of the sum formulae, where . They are useful when we want to find the trigonometric function of an angle that is double a special angle.
- The half-angle formulae are also a special case, and are useful when we want to find the trigonometric function of an angle which is half of a special angle (in other words, ).
- Although each half-angle formula has a sign, the sign that is applied in each case depends on the quadrant the angle falls in and the rules for applying signs to trigonometric functions.
Double-Angle Formulae
In the previous concept, we used addition and subtraction formulae for trigonometric functions. Now, we take another look at those same formulae. The double-angle formulae are a special case of the sum formulae, where . In other words, they allow us to find the trigonometric function of an angle that is double a special angle. Formulae can be derived to find the sine, cosine, and tangent in such cases, and these formulae are useful in simplifying trigonometric expressions. Deriving the double-angle formula for sine begins with the sum formula that was introduced previously: . If we let , then we have: \displaystyle{ \begin{align} \sin(\theta + \theta) &= \sin \theta \cos \theta + \cos \theta \sin \theta \\ \sin(2\theta) &= 2\sin \theta \cos \theta \end{align} } The double-angle formula for cosine can be derived similarly, and is: Notice that we can apply the Pythagorean identities to get two more variations of the cosine formula: \displaystyle{ \begin{align} \cos{\left(2\theta \right)} &= \cos^2 \theta - \sin^2 \theta \\ &= \left(1- \sin^2 \theta \right) - \sin^2 \theta \\ &= 1- 2\sin^2 \theta \end{align} } \displaystyle{ \begin{align} \cos{\left(2\theta\right)} &= \cos^2 \theta - \sin^2 \theta \\ &= \cos^2 \theta - \left(1- \cos^2 \theta \right) \\ &= 2 \cos^2 \theta -1 \end{align} } Similarly, to derive the double-angle formula for tangent, replacing in the sum formula gives \displaystyle{ \begin{align} \tan{\left(\alpha + \beta \right)} &= \frac{ \tan \alpha + \tan \beta}{1 - \tan \alpha \tan \beta} \\ \tan{\left(\theta + \theta \right)} &= \frac{ \tan \theta + \tan \theta}{1 - \tan \theta \tan \theta} \\ \tan{\left(2\theta \right)} &= \frac{ 2\tan \theta }{1 - \tan^2 \theta} \end{align} } The double-angle formulae are summarized as follows:Example
Find using the function . Notice that is a special angle, and is twice its value. We will apply the double-angle formula for sine: . In this case, we let because we want to solve . Substitute into the formula: \displaystyle{ \begin{align} \sin{\left(60^{\circ}\right)} &= \sin{\left(30^{\circ} + 30^{\circ}\right)} \\ &= 2\sin (30^{\circ}) \cos (30^{\circ}) \end{align} } From the unit circle, we can identify that and . Substitute these values into the formula: Simplify: \displaystyle{ \begin{align} \sin{\left(60^{\circ}\right)} &= 2\left(\frac{\sqrt{3}}{4}\right) \\ &= \frac{\sqrt{3}}{2} \end{align} }Half-Angle Formulae
The half-angle formulae can be derived from the double-angle formulae. They are useful for finding the trigonometric function of an angle which is half of a special angle (in other words, ). The half-angle formulae are as follows:Example
Find using a half-angle formula. Recall that is a special angle, and note that . We can thus apply the half-angle formula with : \displaystyle{ \begin{align} \sin{\left(15^{\circ}\right)} &= \sin{\left(\frac{30^{\circ}}{2}\right)} \\ &= \pm \sqrt{\frac{1- \cos(30^{\circ})}{2}} \end{align} } Substitute , which is provided in the unit circle, and simplify: \displaystyle{ \begin{align} \sin{\left(15^{\circ}\right)} &= \sqrt{\frac{1- \frac{\sqrt{3}}{2}}{2}} \\ &= \sqrt{\frac{\frac{2-\sqrt{3}}{2}}{2}} \\ &= \sqrt{\frac{2-\sqrt{3}}{4}} \\ &= \frac{\sqrt{2-\sqrt{3}}}{4} \\ \end{align} } Notice that we used only the positive root because falls in the first quadrant and is therefore positive.Trigonometric Symmetry Identities
The trigonometric symmetry identities are based on principles of even and odd functions that can be observed in their graphs.Learning Objectives
Explain the trigonometric symmetry identities using the graphs of the trigonometric functionsKey Takeaways
Key Points
- Trigonometric functions are either even or odd, meaning that they are symmetric around the -axis or origin, respectively.
- The even trigonometric functions are cosine and secant, and the odd trigonometric functions are sine, cosecant, tangent, and cotangent.
- The definitions of even and odd functions can be used to derive symmetry identities that correspond to each of the six trigonometric functions.
- The symmetry identities can be used to find the trigonometric functions of negative values.
Key Terms
- odd function: A continuous set of points for which , and there is symmetry about the origin.
- even function: A continuous set of points for which , and there is symmetry about the -axis.
Symmetry in Trigonometric Functions
We have previously discussed even and odd functions. Recall that even functions are symmetric about the -axis, and odd functions are symmetric about the origin, . Recall that cosine is an even function because it is symmetric about the -axis. On the other hand, sine and tangent are odd functions because they are symmetric about the origin. We will now consider each of the trigonometric functions and their cofunctions (secant, cosecant, and cotangent), and observe symmetry in their graphs. This symmetry is used to derive certain identities.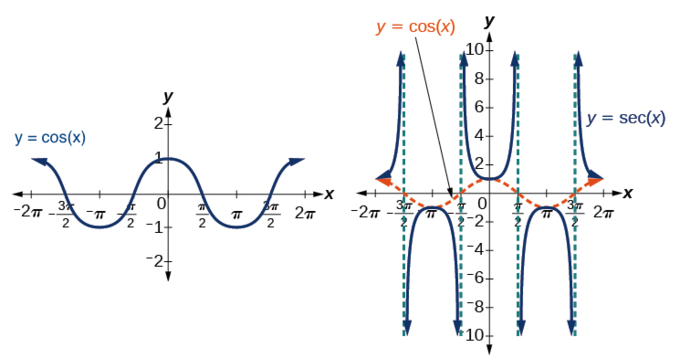 Symmetry around the -axis: Cosine and secant are even functions, with symmetry around the -axis.
Symmetry around the -axis: Cosine and secant are even functions, with symmetry around the -axis.The cosine and secant functions are symmetric about the y-axis. Graphs that are symmetric about the -axis represent even functions. For even functions, any two points with opposite -values have the same function value. This is expressed mathematically as for all in the domain of .
 Symmetry around the origin: Sine, cosecant, tangent, and cotangent are odd functions, and are symmetric around the origin.
Symmetry around the origin: Sine, cosecant, tangent, and cotangent are odd functions, and are symmetric around the origin.The sine, cosecant, tangent, and cotangent functions are symmetric about the origin. Graphs that are symmetric about the origin represent odd functions. For odd functions, any two points with opposite -values also have opposite -values. This is expressed mathematically as for all in the domain of .
